


Next: Visible vs. Infrared
Up: Cirrus Cloud Visible
Previous: Calibration Technique
Return to the Publications.
Return to the Index.
The results of the calibrated, attenuation corrected VIL data between
the heights of 6 to 9 km above the HSRL are seen in Figure 12.
The point comparison between the HSRL 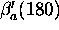 and the calibrated
VIL
and the calibrated
VIL 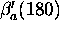 shows more scatter than the comparison for the data between
the heights of 6 and 7.5 km. This was a result of the misalignment errors
between the two profiles described earlier and the usage of a bulk aerosol
backscatter phase function for the correction of the attenuation in the VIL
signal.
shows more scatter than the comparison for the data between
the heights of 6 and 7.5 km. This was a result of the misalignment errors
between the two profiles described earlier and the usage of a bulk aerosol
backscatter phase function for the correction of the attenuation in the VIL
signal.
The lowest 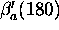 detected by the VIL at a horizontal distance
of 24 km was approximately
3
detected by the VIL at a horizontal distance
of 24 km was approximately
3  10
10 m
m sr
sr (-6.5 in Figure 12).
The VIL data centered around a value of 5.0
(-6.5 in Figure 12).
The VIL data centered around a value of 5.0  10
10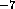 m
m sr
sr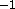 (-6.3) corresponded to non-cirrus aerosol backscatter. Little correlation was
expected in this range because of the wavelength dependence of the scattering
at the two lidar wavelengths by the non-cirrus aerosols. The
(-6.3) corresponded to non-cirrus aerosol backscatter. Little correlation was
expected in this range because of the wavelength dependence of the scattering
at the two lidar wavelengths by the non-cirrus aerosols. The
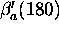 centered around
1.0
centered around
1.0  10
10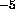 m
m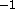 sr
sr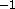 (-5.0)
correspond to the backscatter by cirrus cloud ice particles.
(-5.0)
correspond to the backscatter by cirrus cloud ice particles.
In Figure 12, the VIL calibrated  are
approximately 1.5 times greater than the HSRL
are
approximately 1.5 times greater than the HSRL  . This was
a result of the VIL signal being corrected for multiple scattering
while the HSRL signal was not. If a multiple scattering correction factor
of 0.5 was included in the calculation of the HSRL
. This was
a result of the VIL signal being corrected for multiple scattering
while the HSRL signal was not. If a multiple scattering correction factor
of 0.5 was included in the calculation of the HSRL 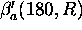 (similar to the VIL multiple scattering correction), then
(similar to the VIL multiple scattering correction), then  in Equation 10 would increase resulting in an
increase in
in Equation 10 would increase resulting in an
increase in  .
.
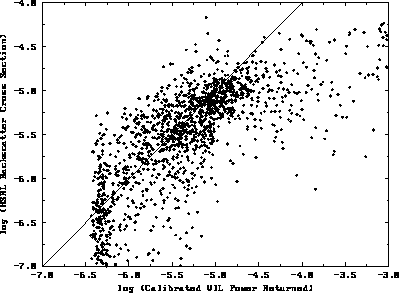
Figure 12: VIL--HSRL cirrus cloud data point comparison on December 1, 1989
from 19:29 to 21:20 GMT. The x-axis is the logarithm of the calibrated VIL
aerosol backscatter cross sections.
The y-axis is the logarithm of the HSRL aerosol backscatter cross sections.
The point comparison is for data between 6 km and 9 km in height,
the depth of the cirrus cloud layer. A one to one line is plotted for
reference.
The calibration of the VIL data was extended to the cross wind scans for
the two time periods. This extension produced aerosol
backscatter cross sections for the mesoscale volume at a resolution of 2-3 km
parallel to the wind (a function of the wind speed and the scanning rate of the
VIL) and a resolution of 60 m in both the cross wind scan direction and
the vertical. To determine whether the VIL calibrated 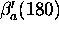 for the mesoscale volume had a similar distribution to the VIL simulated RTI
and HSRL RTI
for the mesoscale volume had a similar distribution to the VIL simulated RTI
and HSRL RTI 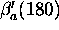 distributions, 50 point histograms of the
data between the heights of 6 km and 11 km were computed.
To create the VIL histograms, the molecular
distributions, 50 point histograms of the
data between the heights of 6 km and 11 km were computed.
To create the VIL histograms, the molecular
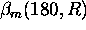 was calculated using Equation 11 (for 1064 nm)
and subtracted from the VIL calibrated backscattered signal.
This allowed for the VIL and HSRL
was calculated using Equation 11 (for 1064 nm)
and subtracted from the VIL calibrated backscattered signal.
This allowed for the VIL and HSRL  distributions for
the atmospheric aerosols to be compared.
distributions for
the atmospheric aerosols to be compared.
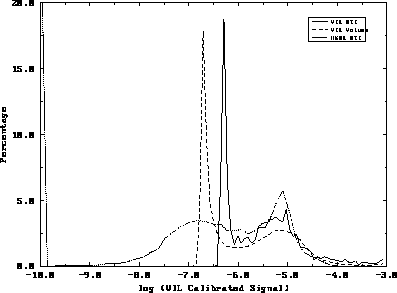
Figure 13: 50 point histograms of  between heights of 6 km and
11 km from 19:29 to 21:20 GMT on December 1, 1989. The x-axis is the
logarithm of
between heights of 6 km and
11 km from 19:29 to 21:20 GMT on December 1, 1989. The x-axis is the
logarithm of  and the y-axis is the percentage of points
falling within each
interval. Histograms of the HSRL
and the y-axis is the percentage of points
falling within each
interval. Histograms of the HSRL 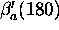 (short dash), vertical
profile of VIL
(short dash), vertical
profile of VIL  over the HSRL position (24 km to the East
of the VIL (solid line)), and the VIL
over the HSRL position (24 km to the East
of the VIL (solid line)), and the VIL 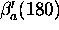 throughout a
mesoscale volume produced from consecutive VIL cross wind scans (long dash)
are shown. The cirrus cloud threshold value used in Section 3 coincides
with a value of 1.0
throughout a
mesoscale volume produced from consecutive VIL cross wind scans (long dash)
are shown. The cirrus cloud threshold value used in Section 3 coincides
with a value of 1.0  10
10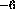 m
m sr
sr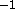 .
.
The resulting histograms are seen in Figure 13.
A peak occurs in all three histograms near
8  10
10 m
m sr
sr (-5.2). This
peak was associated with the cirrus ice particle
(-5.2). This
peak was associated with the cirrus ice particle 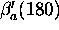 .
In all three histograms, the range of
.
In all three histograms, the range of 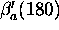 for
the ice crystals spanned from 1
for
the ice crystals spanned from 1  10
10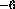 to
1
to
1  10
10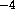 m
m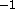 sr
sr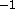 .
The peak at the smaller
.
The peak at the smaller 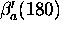 , which
represents the non-cirrus aerosols, occurred near
1.5
, which
represents the non-cirrus aerosols, occurred near
1.5  10
10 m
m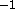 sr
sr for the HSRL RTI and the
VIL data from the mesoscale volume. The non-cirrus aerosol
peak for the VIL RTI, which occurred at
5.0
for the HSRL RTI and the
VIL data from the mesoscale volume. The non-cirrus aerosol
peak for the VIL RTI, which occurred at
5.0  10
10 m
m sr
sr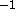 , shows the loss of signal
at far ranges for the VIL. For
, shows the loss of signal
at far ranges for the VIL. For  below
3.8
below
3.8  10
10 m
m sr
sr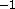 (neglecting attenuation affects),
the VIL receiver was not sensitive enough
to detect the radiation backscattered from a horizontal distance of
24 km. The
(neglecting attenuation affects),
the VIL receiver was not sensitive enough
to detect the radiation backscattered from a horizontal distance of
24 km. The 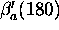 limit of detectability
for a distance of 6 km can be seen in the histogram of the VIL data
for the mesoscale volume. The smallest detectable
limit of detectability
for a distance of 6 km can be seen in the histogram of the VIL data
for the mesoscale volume. The smallest detectable  was
1.25
was
1.25  10
10 m
m sr
sr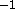 (-6.8) as seen in Figure 13.
Some HSRL
(-6.8) as seen in Figure 13.
Some HSRL 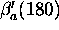 were smaller than
1.0
were smaller than
1.0  10
10 m
m sr
sr . This was a result
of incomplete separation between the aerosol and molecular channels
for regions with small aerosol backscatter. This incomplete
separation even led to some negative HSRL
. This was a result
of incomplete separation between the aerosol and molecular channels
for regions with small aerosol backscatter. This incomplete
separation even led to some negative HSRL  beneath the cirrus
cloud layer. This problem has
been removed in a new configuration of the HSRL (Piironen and Eloranta (1993)).
Figure 13 also shows a relative minimum value for the
beneath the cirrus
cloud layer. This problem has
been removed in a new configuration of the HSRL (Piironen and Eloranta (1993)).
Figure 13 also shows a relative minimum value for the
 near 1.0
near 1.0  10
10 m
m sr
sr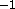 for all three
histograms. This relative minimum shows a clear
separation between the background aerosol backscatter and the ice particle
backscatter. This minimum value coincides with the threshold value used for the
cirrus cloud determination in Section 3. At 1064 nm, the molecular backscatter
cross section at a height of 6 km (calculated from the coincident rawinsonde
density profile) was 6.44
for all three
histograms. This relative minimum shows a clear
separation between the background aerosol backscatter and the ice particle
backscatter. This minimum value coincides with the threshold value used for the
cirrus cloud determination in Section 3. At 1064 nm, the molecular backscatter
cross section at a height of 6 km (calculated from the coincident rawinsonde
density profile) was 6.44  10
10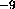 m
m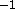 sr
sr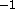 . A typical
aerosol backscatter cross section taken from the cirrus volume was
1.58
. A typical
aerosol backscatter cross section taken from the cirrus volume was
1.58  10
10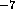 m
m sr
sr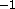 .
By comparing these two values, it was determined that
the signal backscattered by the background aerosols at a wavelength
of 1064
.
By comparing these two values, it was determined that
the signal backscattered by the background aerosols at a wavelength
of 1064 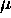 m was at least a factor of twenty greater than the
molecular backscatter at the cirrus cloud heights during this experiment.
m was at least a factor of twenty greater than the
molecular backscatter at the cirrus cloud heights during this experiment.
Although the peak at the ice crystal backscatter cross sections coincides
for the three histograms, large variations are seen in the calibration plot
between the VIL and the HSRL (Figure 12). The scatter
seen in Figure 12 can result from variations in the
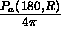 throughout the cirrus mesoscale volume,
misalignments between the two lidar systems, and/or signal noise.
The
throughout the cirrus mesoscale volume,
misalignments between the two lidar systems, and/or signal noise.
The 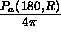 are dependent on the shape (or type)
of the ice crystals scattering the laser light.
The
are dependent on the shape (or type)
of the ice crystals scattering the laser light.
The  for different types of ice crystals
can change by 0.048 sr
for different types of ice crystals
can change by 0.048 sr as calculated by Takano and Liou (1989) (the
values for the ice crystal
as calculated by Takano and Liou (1989) (the
values for the ice crystal  varied between 0.037
and 0.085 sr
varied between 0.037
and 0.085 sr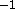 ). If the
cirrus
). If the
cirrus  changed significantly over the volume,
then the actual
changed significantly over the volume,
then the actual 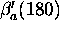 will change along with the
will change along with the 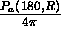 causing errors
in the attenuation correction technique. A second cause for the errors in the
calibration may be a result of the variations of the ice crystal
causing errors
in the attenuation correction technique. A second cause for the errors in the
calibration may be a result of the variations of the ice crystal
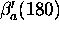 across the cirrus clouds.
across the cirrus clouds.
The variation of the 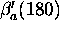 for visible wavelengths
within the cirrus clouds will give an estimate on the allowable error
between the VIL scan plane and the HSRL position. If the
cirrus particles vary significantly from point to point then the alignment
of the VIL has to be precise. If, on the other hand, the
for visible wavelengths
within the cirrus clouds will give an estimate on the allowable error
between the VIL scan plane and the HSRL position. If the
cirrus particles vary significantly from point to point then the alignment
of the VIL has to be precise. If, on the other hand, the 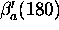 for the cirrus particles vary slowly within the cloud, then small
alignment errors will be acceptable. To determine the variation of
the ice crystal
for the cirrus particles vary slowly within the cloud, then small
alignment errors will be acceptable. To determine the variation of
the ice crystal  between the cirrus cloud data points
in the scanned mesoscale volume, autocorrelations
of the along wind and cross wind cirrus scans were calculated.
between the cirrus cloud data points
in the scanned mesoscale volume, autocorrelations
of the along wind and cross wind cirrus scans were calculated.
During the first time period (18:07-19:24 GMT) scattered cirrus clouds
occurred throughout the mesoscale volume. Along the wind, there
was 95.6% correlation between cirrus cloud  for data
points separated by 100 meters in the horizontal. For a 200 meter
horizontal separation, there was an 89.5% correlation between cirrus
cloud points. At 500 meters, there was a 73.9%
correlation and at a distance of 1000 meters, the correlation
dropped to 55.9%. In the vertical, for data points separated by
60, 120 and 240 meters, there was an 82.9%, 58.9%, and a 31.4% correlation
between the ice crystal
for data
points separated by 100 meters in the horizontal. For a 200 meter
horizontal separation, there was an 89.5% correlation between cirrus
cloud points. At 500 meters, there was a 73.9%
correlation and at a distance of 1000 meters, the correlation
dropped to 55.9%. In the vertical, for data points separated by
60, 120 and 240 meters, there was an 82.9%, 58.9%, and a 31.4% correlation
between the ice crystal 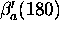 , respectively. The cirrus cloud
, respectively. The cirrus cloud
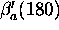 correlations were also calculated for the VIL cross wind scans.
For a 100 meter horizontal separation along the scan plane, there was
a 94.6% correlation. At 200 meters,
an 88.2%, at 500 meters, a 73.2% correlation, and at 1000 meters,
a 54.6% correlation
between the cirrus cloud
correlations were also calculated for the VIL cross wind scans.
For a 100 meter horizontal separation along the scan plane, there was
a 94.6% correlation. At 200 meters,
an 88.2%, at 500 meters, a 73.2% correlation, and at 1000 meters,
a 54.6% correlation
between the cirrus cloud 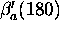 . In the vertical, at a 60 meter
interval, there was a 79.0% correlation, 120 meters,
a 49.1% correlation, and at 240 meter separation, a
. In the vertical, at a 60 meter
interval, there was a 79.0% correlation, 120 meters,
a 49.1% correlation, and at 240 meter separation, a 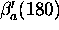 correlation of 29.3% was seen.
correlation of 29.3% was seen.
For this time period, the horizontal and vertical
correlations were similar
for the along and cross wind VIL scans. Good correlation existed for
points separated by 100 to 200 meters. When the length of the correlation
was extended to 500 and 1000 meters, the variation between data points
became significant. The high correlation at 100 and 200 meter separations
in the horizontal suggest that small azimuth angle alignment errors
between the two
systems can be tolerated. If the misalignments between the two profiles
was as large as 1000 meters, then the resulting calibration would be very poor.
In the vertical, only a maximum error of 60 m could be tolerated
when generating a calibration for the VIL.
The vertical cirrus cloud 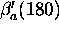 correlations changed more rapidly
than the horizontal correlations. This was due to the vertical wind shear
within the cirrus cloud. For the first time period, the larger
separation lengths had correlations less than 50% for
the cirrus ice crystal
correlations changed more rapidly
than the horizontal correlations. This was due to the vertical wind shear
within the cirrus cloud. For the first time period, the larger
separation lengths had correlations less than 50% for
the cirrus ice crystal 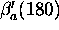 . A calibration for the VIL could
not be produced for larger separation lengths in the horizontal and the
vertical due to the cirrus cloud
. A calibration for the VIL could
not be produced for larger separation lengths in the horizontal and the
vertical due to the cirrus cloud  variations.
variations.
For the second time period (19:29-21:20 GMT), the cirrus clouds were
more spatially uniform due to the presence of a cirrus cloud deck.
For the along wind scan,
at 100, 200, 500, and 1000 meter separations, correlations of 94.8%, 89.3%,
78.6%, and 67.9% were seen, respectively. In the vertical, at 60, 120, and
240 meter data point separations, correlations of
84.2%, 64.4%, and 45.3% were seen,
respectively. For the cross wind scan, at 100, 200, 500, and 1000 meter
separation between points, correlations of 95.2%, 89.3%, 75.6%,
and 59.4% between the cloud data points existed. In the vertical, for
60 meter data point
separation, an 82.1% correlation was seen while at 120 meters a 59.0%
correlation occurred. At a 240 meter separation in the vertical,
a correlation of
36.1% was detected. During this time period, the correlation values
along the wind were higher than those in the cross wind scans for
the larger distances between data points. This was a result of the widespread
cirrus cloud deck over most of the region at this time which had
an aspect ratio of 9:1.
For the VIL RTI simulating the HSRL RTI during the first time period,
the correlation between cloud points was calculated. The correlation was
computed along the wind direction with a separation between points (scans) in
the horizontal of approximately 3 km and
with a 60 meter data point separation in the vertical.
In the horizontal, correlations of 76.3%, 48.2%, 18.1%,
and 15.5% were seen at 1, 2, 5, and 10 scan separations (points).
In the vertical, correlations of 89.2%, 71.0%, and 52.7% were seen
at 60, 120, and 240 meter separations, respectively.
For the second time period, correlations of
83.3%, 65.6%, 46.9%, and 36.1% were seen for 1, 2, 5, and 10
scan separations respectively. In the vertical, separations of 60,
120, and 240 meters resulted in 88.9%, 73.3%, and 55.1% correlations between
the cirrus cloud aerosol backscatter cross sections, respectively.
Higher correlations were seen in the vertical because these points were
taken from a single cirrus cloud scan while the horizontal
data points were taken from consecutive scans.
The vertical correlations were similar to the previously calculated values as
would be expected. There was very little correlation between
the cirrus cloud particulate  in the horizontal because of the
large distances separating consecutive data points and the 2 km averaging
along the scans to produce each profile. The second time period had
higher correlations in the horizontal than the first time period. This was a
result of a widespread cirrus cloud deck which occurred during the second
time period
while the earlier period had scattered cirrus clouds throughout the region.
The scattered cirrus clouds created a situation which made it difficult to
produce a VIL RTI to match the HSRL RTI. The cirrus cloud deck on the
other hand had more uniformity which allowed for greater scan angle
errors between the two systems.
Also, the VIL scan plane was more closely aligned with the wind direction
at the cirrus cloud heights during the later period.
in the horizontal because of the
large distances separating consecutive data points and the 2 km averaging
along the scans to produce each profile. The second time period had
higher correlations in the horizontal than the first time period. This was a
result of a widespread cirrus cloud deck which occurred during the second
time period
while the earlier period had scattered cirrus clouds throughout the region.
The scattered cirrus clouds created a situation which made it difficult to
produce a VIL RTI to match the HSRL RTI. The cirrus cloud deck on the
other hand had more uniformity which allowed for greater scan angle
errors between the two systems.
Also, the VIL scan plane was more closely aligned with the wind direction
at the cirrus cloud heights during the later period.
The cirrus cloud  correlations revealed the types of error
which would occur due to misalignments between the viewing positions of
the two lidars. If large scan angle errors were present (greater than 1
correlations revealed the types of error
which would occur due to misalignments between the viewing positions of
the two lidars. If large scan angle errors were present (greater than 1 ),
then the cirrus cloud point comparison between the HSRL RTI and the VIL
simulated RTI would be poor due to the variation of the
),
then the cirrus cloud point comparison between the HSRL RTI and the VIL
simulated RTI would be poor due to the variation of the 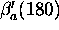 within the cirrus clouds. For acceptable calibration results, the
VIL azimuth angle errors have to be less than 0.5
within the cirrus clouds. For acceptable calibration results, the
VIL azimuth angle errors have to be less than 0.5 and the elevation
angle errors have to be less than 0.25
and the elevation
angle errors have to be less than 0.25 .
.
For the two time periods, the cirrus cloud  correlation values were similar but the
calibration plots were not. The attempted calibration plot for the first time
period (18:08 to 19:24 GMT) was very poor and as a result was not shown.
The difference between the two time periods
was the direction of the cirrus cloud advection and the widespread
cirrus cloud deck throughout the later period. The clouds
during the first time period were advected into the region from 283
correlation values were similar but the
calibration plots were not. The attempted calibration plot for the first time
period (18:08 to 19:24 GMT) was very poor and as a result was not shown.
The difference between the two time periods
was the direction of the cirrus cloud advection and the widespread
cirrus cloud deck throughout the later period. The clouds
during the first time period were advected into the region from 283 while
the clouds during the second time period came from 277
while
the clouds during the second time period came from 277 (on average). This
difference of 6
(on average). This
difference of 6 between the wind direction and the
VIL along wind scan direction result in point comparison errors in the
calibration plot. The errors occurred when the VIL data was averaged
along the scan plane to simulate the HSRL data (which was averaged along the
wind direction). Since the
VIL was averaged over the same distance as the HSRL profile (2 km), the
offset between the VIL and HSRL data points at the end of
the averaging length (1 km from each system)
was 200 meters for the later time period and 300 meters
for the early time period. The aerosol backscatter cross section correlation
values across the wind dropped approximately 14% at the end of the averaging
distances for the given time periods. This reduction in correlation, along
with the scattered cirrus clouds, made the
production of a VIL calibration plot from the first time period data
impossible. This shows
the importance of either aligning the VIL along the wind or using smaller
averaging times in determining the cirrus cloud visible optical properties
from the HSRL.
between the wind direction and the
VIL along wind scan direction result in point comparison errors in the
calibration plot. The errors occurred when the VIL data was averaged
along the scan plane to simulate the HSRL data (which was averaged along the
wind direction). Since the
VIL was averaged over the same distance as the HSRL profile (2 km), the
offset between the VIL and HSRL data points at the end of
the averaging length (1 km from each system)
was 200 meters for the later time period and 300 meters
for the early time period. The aerosol backscatter cross section correlation
values across the wind dropped approximately 14% at the end of the averaging
distances for the given time periods. This reduction in correlation, along
with the scattered cirrus clouds, made the
production of a VIL calibration plot from the first time period data
impossible. This shows
the importance of either aligning the VIL along the wind or using smaller
averaging times in determining the cirrus cloud visible optical properties
from the HSRL.



Next: Visible vs. Infrared
Up: Cirrus Cloud Visible
Previous: Calibration Technique
Return to the Publications.
Return to the Index.
Antti Piironen
Thu Apr 11 08:27:54 CDT 1996
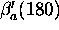 and the calibrated
VIL
and the calibrated
VIL 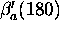 shows more scatter than the comparison for the data between
the heights of 6 and 7.5 km. This was a result of the misalignment errors
between the two profiles described earlier and the usage of a bulk aerosol
backscatter phase function for the correction of the attenuation in the VIL
signal.
shows more scatter than the comparison for the data between
the heights of 6 and 7.5 km. This was a result of the misalignment errors
between the two profiles described earlier and the usage of a bulk aerosol
backscatter phase function for the correction of the attenuation in the VIL
signal.



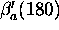 detected by the VIL at a horizontal distance
of 24 km was approximately
3
detected by the VIL at a horizontal distance
of 24 km was approximately
3  10
10 m
m sr
sr (-6.5 in Figure
(-6.5 in Figure  10
10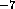 m
m sr
sr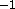 (-6.3) corresponded to non-cirrus aerosol backscatter. Little correlation was
expected in this range because of the wavelength dependence of the scattering
at the two lidar wavelengths by the non-cirrus aerosols. The
(-6.3) corresponded to non-cirrus aerosol backscatter. Little correlation was
expected in this range because of the wavelength dependence of the scattering
at the two lidar wavelengths by the non-cirrus aerosols. The
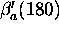 centered around
1.0
centered around
1.0  10
10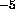 m
m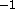 sr
sr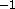 (-5.0)
correspond to the backscatter by cirrus cloud ice particles.
(-5.0)
correspond to the backscatter by cirrus cloud ice particles.
 are
approximately 1.5 times greater than the HSRL
are
approximately 1.5 times greater than the HSRL  . This was
a result of the VIL signal being corrected for multiple scattering
while the HSRL signal was not. If a multiple scattering correction factor
of 0.5 was included in the calculation of the HSRL
. This was
a result of the VIL signal being corrected for multiple scattering
while the HSRL signal was not. If a multiple scattering correction factor
of 0.5 was included in the calculation of the HSRL 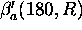 (similar to the VIL multiple scattering correction), then
(similar to the VIL multiple scattering correction), then  in Equation
in Equation  .
.

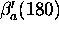 for the mesoscale volume had a similar distribution to the VIL simulated RTI
and HSRL RTI
for the mesoscale volume had a similar distribution to the VIL simulated RTI
and HSRL RTI 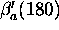 distributions, 50 point histograms of the
data between the heights of 6 km and 11 km were computed.
To create the VIL histograms, the molecular
distributions, 50 point histograms of the
data between the heights of 6 km and 11 km were computed.
To create the VIL histograms, the molecular
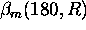 was calculated using Equation
was calculated using Equation  distributions for
the atmospheric aerosols to be compared.
distributions for
the atmospheric aerosols to be compared.

 between heights of 6 km and
11 km from 19:29 to 21:20 GMT on December 1, 1989. The x-axis is the
logarithm of
between heights of 6 km and
11 km from 19:29 to 21:20 GMT on December 1, 1989. The x-axis is the
logarithm of  and the y-axis is the percentage of points
falling within each
interval. Histograms of the HSRL
and the y-axis is the percentage of points
falling within each
interval. Histograms of the HSRL 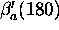 (short dash), vertical
profile of VIL
(short dash), vertical
profile of VIL  over the HSRL position (24 km to the East
of the VIL (solid line)), and the VIL
over the HSRL position (24 km to the East
of the VIL (solid line)), and the VIL 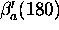 throughout a
mesoscale volume produced from consecutive VIL cross wind scans (long dash)
are shown. The cirrus cloud threshold value used in Section 3 coincides
with a value of 1.0
throughout a
mesoscale volume produced from consecutive VIL cross wind scans (long dash)
are shown. The cirrus cloud threshold value used in Section 3 coincides
with a value of 1.0  10
10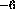 m
m sr
sr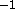 .
. 10
10 m
m sr
sr (-5.2). This
peak was associated with the cirrus ice particle
(-5.2). This
peak was associated with the cirrus ice particle 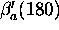 .
In all three histograms, the range of
.
In all three histograms, the range of 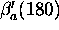 for
the ice crystals spanned from 1
for
the ice crystals spanned from 1  10
10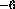 to
1
to
1  10
10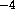 m
m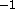 sr
sr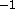 .
The peak at the smaller
.
The peak at the smaller 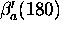 , which
represents the non-cirrus aerosols, occurred near
1.5
, which
represents the non-cirrus aerosols, occurred near
1.5  10
10 m
m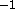 sr
sr for the HSRL RTI and the
VIL data from the mesoscale volume. The non-cirrus aerosol
peak for the VIL RTI, which occurred at
5.0
for the HSRL RTI and the
VIL data from the mesoscale volume. The non-cirrus aerosol
peak for the VIL RTI, which occurred at
5.0  10
10 m
m sr
sr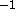 , shows the loss of signal
at far ranges for the VIL. For
, shows the loss of signal
at far ranges for the VIL. For  below
3.8
below
3.8  10
10 m
m sr
sr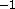 (neglecting attenuation affects),
the VIL receiver was not sensitive enough
to detect the radiation backscattered from a horizontal distance of
24 km. The
(neglecting attenuation affects),
the VIL receiver was not sensitive enough
to detect the radiation backscattered from a horizontal distance of
24 km. The 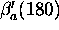 limit of detectability
for a distance of 6 km can be seen in the histogram of the VIL data
for the mesoscale volume. The smallest detectable
limit of detectability
for a distance of 6 km can be seen in the histogram of the VIL data
for the mesoscale volume. The smallest detectable  was
1.25
was
1.25  10
10 m
m sr
sr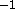 (-6.8) as seen in Figure
(-6.8) as seen in Figure 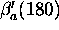 were smaller than
1.0
were smaller than
1.0  10
10 m
m sr
sr . This was a result
of incomplete separation between the aerosol and molecular channels
for regions with small aerosol backscatter. This incomplete
separation even led to some negative HSRL
. This was a result
of incomplete separation between the aerosol and molecular channels
for regions with small aerosol backscatter. This incomplete
separation even led to some negative HSRL  beneath the cirrus
cloud layer. This problem has
been removed in a new configuration of the HSRL (Piironen and Eloranta (1993)).
Figure
beneath the cirrus
cloud layer. This problem has
been removed in a new configuration of the HSRL (Piironen and Eloranta (1993)).
Figure  near 1.0
near 1.0  10
10 m
m sr
sr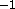 for all three
histograms. This relative minimum shows a clear
separation between the background aerosol backscatter and the ice particle
backscatter. This minimum value coincides with the threshold value used for the
cirrus cloud determination in Section 3. At 1064 nm, the molecular backscatter
cross section at a height of 6 km (calculated from the coincident rawinsonde
density profile) was 6.44
for all three
histograms. This relative minimum shows a clear
separation between the background aerosol backscatter and the ice particle
backscatter. This minimum value coincides with the threshold value used for the
cirrus cloud determination in Section 3. At 1064 nm, the molecular backscatter
cross section at a height of 6 km (calculated from the coincident rawinsonde
density profile) was 6.44  10
10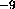 m
m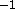 sr
sr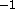 . A typical
aerosol backscatter cross section taken from the cirrus volume was
1.58
. A typical
aerosol backscatter cross section taken from the cirrus volume was
1.58  10
10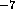 m
m sr
sr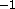 .
By comparing these two values, it was determined that
the signal backscattered by the background aerosols at a wavelength
of 1064
.
By comparing these two values, it was determined that
the signal backscattered by the background aerosols at a wavelength
of 1064 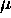 m was at least a factor of twenty greater than the
molecular backscatter at the cirrus cloud heights during this experiment.
m was at least a factor of twenty greater than the
molecular backscatter at the cirrus cloud heights during this experiment.
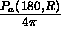 throughout the cirrus mesoscale volume,
misalignments between the two lidar systems, and/or signal noise.
The
throughout the cirrus mesoscale volume,
misalignments between the two lidar systems, and/or signal noise.
The 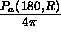 are dependent on the shape (or type)
of the ice crystals scattering the laser light.
The
are dependent on the shape (or type)
of the ice crystals scattering the laser light.
The  for different types of ice crystals
can change by 0.048 sr
for different types of ice crystals
can change by 0.048 sr as calculated by Takano and Liou (1989) (the
values for the ice crystal
as calculated by Takano and Liou (1989) (the
values for the ice crystal  varied between 0.037
and 0.085 sr
varied between 0.037
and 0.085 sr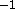 ). If the
cirrus
). If the
cirrus  changed significantly over the volume,
then the actual
changed significantly over the volume,
then the actual 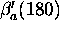 will change along with the
will change along with the 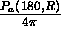 causing errors
in the attenuation correction technique. A second cause for the errors in the
calibration may be a result of the variations of the ice crystal
causing errors
in the attenuation correction technique. A second cause for the errors in the
calibration may be a result of the variations of the ice crystal
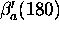 across the cirrus clouds.
across the cirrus clouds.
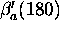 for visible wavelengths
within the cirrus clouds will give an estimate on the allowable error
between the VIL scan plane and the HSRL position. If the
cirrus particles vary significantly from point to point then the alignment
of the VIL has to be precise. If, on the other hand, the
for visible wavelengths
within the cirrus clouds will give an estimate on the allowable error
between the VIL scan plane and the HSRL position. If the
cirrus particles vary significantly from point to point then the alignment
of the VIL has to be precise. If, on the other hand, the 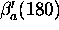 for the cirrus particles vary slowly within the cloud, then small
alignment errors will be acceptable. To determine the variation of
the ice crystal
for the cirrus particles vary slowly within the cloud, then small
alignment errors will be acceptable. To determine the variation of
the ice crystal  between the cirrus cloud data points
in the scanned mesoscale volume, autocorrelations
of the along wind and cross wind cirrus scans were calculated.
between the cirrus cloud data points
in the scanned mesoscale volume, autocorrelations
of the along wind and cross wind cirrus scans were calculated.
 for data
points separated by 100 meters in the horizontal. For a 200 meter
horizontal separation, there was an 89.5% correlation between cirrus
cloud points. At 500 meters, there was a 73.9%
correlation and at a distance of 1000 meters, the correlation
dropped to 55.9%. In the vertical, for data points separated by
60, 120 and 240 meters, there was an 82.9%, 58.9%, and a 31.4% correlation
between the ice crystal
for data
points separated by 100 meters in the horizontal. For a 200 meter
horizontal separation, there was an 89.5% correlation between cirrus
cloud points. At 500 meters, there was a 73.9%
correlation and at a distance of 1000 meters, the correlation
dropped to 55.9%. In the vertical, for data points separated by
60, 120 and 240 meters, there was an 82.9%, 58.9%, and a 31.4% correlation
between the ice crystal 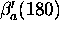 , respectively. The cirrus cloud
, respectively. The cirrus cloud
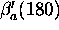 correlations were also calculated for the VIL cross wind scans.
For a 100 meter horizontal separation along the scan plane, there was
a 94.6% correlation. At 200 meters,
an 88.2%, at 500 meters, a 73.2% correlation, and at 1000 meters,
a 54.6% correlation
between the cirrus cloud
correlations were also calculated for the VIL cross wind scans.
For a 100 meter horizontal separation along the scan plane, there was
a 94.6% correlation. At 200 meters,
an 88.2%, at 500 meters, a 73.2% correlation, and at 1000 meters,
a 54.6% correlation
between the cirrus cloud 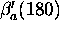 . In the vertical, at a 60 meter
interval, there was a 79.0% correlation, 120 meters,
a 49.1% correlation, and at 240 meter separation, a
. In the vertical, at a 60 meter
interval, there was a 79.0% correlation, 120 meters,
a 49.1% correlation, and at 240 meter separation, a 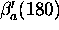 correlation of 29.3% was seen.
correlation of 29.3% was seen.
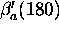 correlations changed more rapidly
than the horizontal correlations. This was due to the vertical wind shear
within the cirrus cloud. For the first time period, the larger
separation lengths had correlations less than 50% for
the cirrus ice crystal
correlations changed more rapidly
than the horizontal correlations. This was due to the vertical wind shear
within the cirrus cloud. For the first time period, the larger
separation lengths had correlations less than 50% for
the cirrus ice crystal 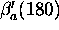 . A calibration for the VIL could
not be produced for larger separation lengths in the horizontal and the
vertical due to the cirrus cloud
. A calibration for the VIL could
not be produced for larger separation lengths in the horizontal and the
vertical due to the cirrus cloud  variations.
variations.
 in the horizontal because of the
large distances separating consecutive data points and the 2 km averaging
along the scans to produce each profile. The second time period had
higher correlations in the horizontal than the first time period. This was a
result of a widespread cirrus cloud deck which occurred during the second
time period
while the earlier period had scattered cirrus clouds throughout the region.
The scattered cirrus clouds created a situation which made it difficult to
produce a VIL RTI to match the HSRL RTI. The cirrus cloud deck on the
other hand had more uniformity which allowed for greater scan angle
errors between the two systems.
Also, the VIL scan plane was more closely aligned with the wind direction
at the cirrus cloud heights during the later period.
in the horizontal because of the
large distances separating consecutive data points and the 2 km averaging
along the scans to produce each profile. The second time period had
higher correlations in the horizontal than the first time period. This was a
result of a widespread cirrus cloud deck which occurred during the second
time period
while the earlier period had scattered cirrus clouds throughout the region.
The scattered cirrus clouds created a situation which made it difficult to
produce a VIL RTI to match the HSRL RTI. The cirrus cloud deck on the
other hand had more uniformity which allowed for greater scan angle
errors between the two systems.
Also, the VIL scan plane was more closely aligned with the wind direction
at the cirrus cloud heights during the later period.
 correlations revealed the types of error
which would occur due to misalignments between the viewing positions of
the two lidars. If large scan angle errors were present (greater than 1
correlations revealed the types of error
which would occur due to misalignments between the viewing positions of
the two lidars. If large scan angle errors were present (greater than 1 ),
then the cirrus cloud point comparison between the HSRL RTI and the VIL
simulated RTI would be poor due to the variation of the
),
then the cirrus cloud point comparison between the HSRL RTI and the VIL
simulated RTI would be poor due to the variation of the 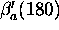 within the cirrus clouds. For acceptable calibration results, the
VIL azimuth angle errors have to be less than 0.5
within the cirrus clouds. For acceptable calibration results, the
VIL azimuth angle errors have to be less than 0.5 and the elevation
angle errors have to be less than 0.25
and the elevation
angle errors have to be less than 0.25 .
.
 correlation values were similar but the
calibration plots were not. The attempted calibration plot for the first time
period (18:08 to 19:24 GMT) was very poor and as a result was not shown.
The difference between the two time periods
was the direction of the cirrus cloud advection and the widespread
cirrus cloud deck throughout the later period. The clouds
during the first time period were advected into the region from 283
correlation values were similar but the
calibration plots were not. The attempted calibration plot for the first time
period (18:08 to 19:24 GMT) was very poor and as a result was not shown.
The difference between the two time periods
was the direction of the cirrus cloud advection and the widespread
cirrus cloud deck throughout the later period. The clouds
during the first time period were advected into the region from 283 while
the clouds during the second time period came from 277
while
the clouds during the second time period came from 277 (on average). This
difference of 6
(on average). This
difference of 6 between the wind direction and the
VIL along wind scan direction result in point comparison errors in the
calibration plot. The errors occurred when the VIL data was averaged
along the scan plane to simulate the HSRL data (which was averaged along the
wind direction). Since the
VIL was averaged over the same distance as the HSRL profile (2 km), the
offset between the VIL and HSRL data points at the end of
the averaging length (1 km from each system)
was 200 meters for the later time period and 300 meters
for the early time period. The aerosol backscatter cross section correlation
values across the wind dropped approximately 14% at the end of the averaging
distances for the given time periods. This reduction in correlation, along
with the scattered cirrus clouds, made the
production of a VIL calibration plot from the first time period data
impossible. This shows
the importance of either aligning the VIL along the wind or using smaller
averaging times in determining the cirrus cloud visible optical properties
from the HSRL.
between the wind direction and the
VIL along wind scan direction result in point comparison errors in the
calibration plot. The errors occurred when the VIL data was averaged
along the scan plane to simulate the HSRL data (which was averaged along the
wind direction). Since the
VIL was averaged over the same distance as the HSRL profile (2 km), the
offset between the VIL and HSRL data points at the end of
the averaging length (1 km from each system)
was 200 meters for the later time period and 300 meters
for the early time period. The aerosol backscatter cross section correlation
values across the wind dropped approximately 14% at the end of the averaging
distances for the given time periods. This reduction in correlation, along
with the scattered cirrus clouds, made the
production of a VIL calibration plot from the first time period data
impossible. This shows
the importance of either aligning the VIL along the wind or using smaller
averaging times in determining the cirrus cloud visible optical properties
from the HSRL.